Enantiomers, Diastereomers & Meso Compounds

Session Objectives

By the end of this
session, students will be able to:

• Explain configuration of enantiomers

• Classify Enantiomers and Diastereomers

• Assign D and L system to isomers

• Define meso compounds

Optical activity

• A solution of optically active molecule (enantiomer) is
placed in a sample tube, plane-polarized light is passed through the tube and a
rotation of the polarization plane takes place

• The light then goes through a second polarizer called an
analyser

• By rotating the analyser until the light passes through
it, the new plane of polarization can be found, and the extent of rotation that
has taken place can be measured

• A mixture of enantiomers with the same amount of each is
called a racemic mixture

• Racemic mixtures are optically inactive (i.e. they cancel
each other out) and are denoted by (±)

Note: the amount
of rotation depends on sample concentration and sample path length

• To obtain a meaningful optical rotation data, we have to
choose standard conditions

• The specific rotation of a compound, designated as [α]_D, is defined as the
observed rotation, when the sample path length l is 1 dm, the sample
concentration C is 1g/mL and light of 599.6 nm wavelength (the D line of a
sodium lamp, which is the yellow light emitted from common sodium lamps) is
mostly used

• As the specific rotation also depends on temperature, the
temperature at which the rotation is measured and denoted more precisely as

Enantiomers can be described as (+) or (-)

• We can use the fact that two enantiomers rotate
plane-polarized light in opposite directions to assign each a label that
doesn’t depend on knowing its configuration

• We call the enantiomer that rotates plane-polarized light
to the right (gives a positive rotation) the (+)-enantiomer (or the dextrorotatory enantiomer)

• And the enantiomer that rotates plane-polarized light to
the left (gives a negative rotation) the (–)-enantiomer (or the laevorotatory enantiomer)

Enantiomers and Diastereomers

• In general molecule with n chiral centers, the maximum
number of stereoisomers possible is 2ⁿ

• That means for one chiral center its 2, for 2 its 4, for 3
its 8 and so forth

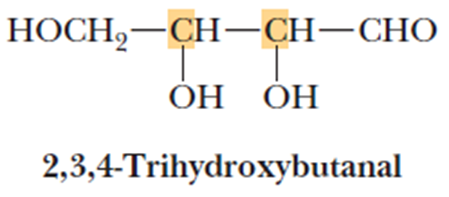
• Let us consider 2,3,4-trihydroxybutanal

• In general molecule with n chiral centers, the maximum
number of stereoisomers possible is 2ⁿ

• Let us consider 2,3,4-trihydroxybutanal

• It contains two chiral centers and 4 stereoisomers are
possible

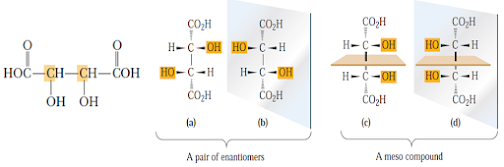
• Stereoisomers (a) and (b) are nonsuperposable mirror
images and are, therefore, a pair of enantiomers

• Stereoisomers (c) and (d) are also nonsuperposable mirror
images and are a second pair of enantiomers

• On naming, enantiomers (a) and (b) as (2R,3R)-erythrose
and (2S,3S)-erythrose; enantiomers (c) and (D) as (2R,3S)-threose and (2S,3R)-threose

• Both belongs to the class of carbohydrates and erythrose
is present in erythrocytes (red blood cells)

• What is the relationship between (a) and (c), (a) and (d),
(b) and (c), (b) and (d)?? Answer is diastereomers

• Diastereomers are the stereoisomers that are not mirror
images

• Molecules with at least two chiral centers can have
diastereomers

Meso compounds

• Molecules containing two or more chiral centers have
special symmetry properties that reduce the number of stereoisomers to fewer
than the maximum number predicted by the 2ⁿ rule

• For example, 2,3-dihydroxybutanedioic acid commonly called
as tartaric acid

• In tartaric acid, carbons 2 and 3 are chiral centers,
number of stereoisomers possible is 4

• (a) and (b) are nonsuperposable mirror images, enantiomers

• (c) and (d) are superposable mirror images, meso compounds

• Also (c) has plane of symmetry and is achiral

• A meso compound contains two or more chiral centers and is
achiral

• To be a meso compound, molecule must also have chiral
isomers

• Hence, tartaric acid has three stereosisomers, one pair of
enantiomers and a meso compound

• Enantiomers of tartaric acid will have same melting point,
boiling point, solubility in water and other common solvents, same value of pKa,
and they undergo the same acid-base reactions

• But differ in optical activity

• Diastereomers have different physical and chemical
properties, even in achiral environments

• Meso tartaric acid has different physical properties from
those of the enantiomers and can be separated from them by methods such as
crystallization

Physical properties of tartaric acid

Summary

• Enantiomer that rotates plane-polarized light to the right
(gives a positive rotation) the (+)-enantiomer (or the dextro-rotatory enantiomer)

• Enantiomer that rotates plane-polarized light to the left
(gives a negative rotation) the (–)-enantiomer (or the laevo-rotatory
enantiomer)

• The direction in which light is rotated is not dependent
on whether a stereogenic centre is R or S

• Optical activity does not tell us the actual configuration
of an enantiomer

• In general molecule with n chiral centers, the maximum
number of stereoisomers possible is 2n

• Diastereomers are the stereoisomers that are not mirror
images

• Molecules with at least two chiral centers can have
diastereomers

• A meso compound contains two or more chiral centers and is
achiral