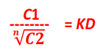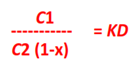Distribution Law
Learning Objectives
• At the end of this lecture, student will be able to
– Define distribution law
– Explain the concept of distribution law
– Compare solubility and distribution law
– Discuss the limitations of distribution law
– Define Henry’s law
– Explain the various applications of distribution law
STATEMENT OF NERNST’S DISTRIBUTION LAW
Nernst (1891) studied the distribution of several solutes
between different appropriate pairs of solvents. He gave a generalization which governs the distribution of a solute between two non-miscible solvents. This is called Nernst’s Distribution law (or Nernst’s Partition law) or simply Distribution law or Partition law.
Definition:
If two immiscible solvents A and B are taken in a beaker,
they form separate layers. When a solute X which is soluble in both solvents is added, it gets distributed or partitioned between them. Molecules of X pass from solvent A to B and from solvent B to A. Finally a dynamic equilibrium is set up. At equilibrium, the rate, at which molecules of X pass from one solvent to the other is balanced
Distribution of solute X between solvent A and B.
Concentration of X in A = constant
Concentration of X in B
Formula
If a solute X distributes itself between two immiscible
solvents A and B at constant temperature and X is in the same molecular condition in both solvents,
If C1 denotes the concentration of the solute in solvent A and C2 the concentration in solvent B,
C1
—–
= KD
C2
The constant KD (or K) is called the Distribution coefficient or Partition coefficient or Distribution ratio
SOLUBILITIES AND DISTRIBUTION LAW
When a solute is shaken with two non-miscible solvents, atbequilibrium both the solvents are saturated with the solute. Since the solubility also represents concentration, distribution law can be written as
C1/C2 = S1/S2 =KD
where S1 and S2 are the solubilities of the solute in the two solvents.
Hence knowing the value of the Distribution coefficient (KD) and the solubility of solute in one of the solvents, the solubility of solute in the second solvent can be calculated.
EXPLANATION OF DISTRIBUTION LAW
• This is an equilibrium law.
• When the distribution of the solute X has reached dynamic equilibrium, the rate (R1) at which molecules of X pass from solvent A to B is proportional to its concentration (C1) in A. The rate (R2) at which molecules of X pass from solvent B to A is proportional to its concentration (C2) in B.
• At equilibrium, the rate of migration of solute from one
solvent to the other is equal.
R1 µ C1 or R1 = k1 X C1
where k1 is a constant
R2 µ C2 or R2 = k2 X C2 where k2 is a constant
Since at equilibrium R1 = R2
k1 × C1 = k2 × C2
C1 = k 2
—- —- = KD
C2 k1
This is the Nernst’s Distribution law equation. Since k1 and k2 are constants at the same temperature, the distribution coefficient KD is also constant if temperature is fixed
LIMITATIONS OF DISTRIBUTION LAW
• Constant temperature – The temperature is kept constant throughout the experiment.
• Same molecular state – The molecular state of the solute is the same in the two solvents. The law does not hold if there is association or dissociation of the solute in one of the solvents.
• Equilibrium concentrations – The concentrations of the solute are noted after the equilibrium has been established.
• Dilute solutions – The concentration of the solute in the two solvents is low. The law does not hold when the concentrations are high.
• Non-miscibility of solvents – The two solvents are non-miscible or only slightly soluble in each other. The extent of mutual solubility of the solvents remains unaltered by the addition of solute to them.
MODIFIED DISTRIBUTION LAW BY CHANGE IN MOLECULAR STATE
• If C1/C2 is constant only if the solute exists as simple
molecules in the two solvents.
• If the solute undergoes association or dissociation in one
of the solvents, it is found that C1/C2 is not constant.
• In these cases, distribution law applies only to that part of the solute which is present as simple molecules.
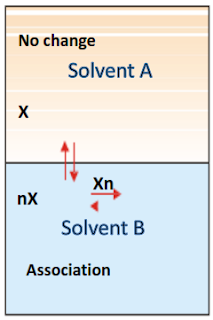
1. When Solute undergoes Association
Suppose the solute is present as simple molecules X In
solvent A. In solvent B, n molecules of X associate to form Xn molecules. Assuming that a few single molecules X are
also present in solvent B the equilibria that exist in the two solvents,
Let C1 be concentration of X in solvent A,
C2 be concentration of Xn in solvent B,
C3 be concentration of X in solvent B
Applying distribution law to the equilibrium
X in solvent A X in solvent B
C1
—- = KD (1)
C3
2. When Solute undergoes Dissociation
Suppose the solute is present as normal molecules X in
solvent A and it dissociates into A + B in solvent B. The equilibria set up in the two solvents
Let C1 be the concentration of X in solvent A and C2 the total concentration of X (dissociated and undissociated) in solvent B.
If the degree of dissociation in solvent B is x,
X A + B
1 – x x x
Hence the concentration of the undissociated (or normal)
molecules in solvent B is C2 (1 – x).
Dissociation
Applying distribution law to normal molecules in the two
solvents
C1
———– = KD
C2 (1-x)
• This is the modified distribution law equation when there
is dissociation in one of the solvents.
• A case of this type arises in the distribution of a weak
acid (e.g., succinic acid or oxalic acid) between ether and water.
• C1 and C2 can be determined by direct titration of the two
layers against standard alkali solution.
• The value of x can be found by measuring electrical conductance of solution in solvent B.
HENRY’S LAW – A FORM OF DISTRIBUTION LAW
Henry’s law states: at a constant temperature the solubility of a gas in a liquid is proportional to the pressure of the gas above it.
It is mathematically expressed as
C = kP
Where C is the solubility (or concentration), k is a constant, and P is the pressure of the gas, k is called Henry’s constant.
• Henry’s law is a form of Distribution law.
• If a vessel containing a liquid and a gas is shaken, at equilibrium the gas can be regarded as distributed between the liquid (Phase A) and the space above (Phase B). Eg. Carbonated beverages
• Henry’s law holds good for dilute solutions of gases which
do not react with the solvent.
• If a mixture of gases is in contact with a liquid, the partial pressure of an individual gas, not their total pressure, determines the mass of each gas dissolving.
• In such a case, the solubility of each gas is proportional
to its partial pressure.
DETERMINATION OF EQUILIBRIUM CONSTANT FROM DISTRIBUTION COEFFICIENT
• Partition co-efficient of Iodine between water and carbon
tetrachloride
• Partition co-efficient of benzoic acid between water and
benzene
APPLICATIONS OF DISTIBUTION LAW
1. EXTRACTION WITH A SOLVENT
• The extraction (removal by a solvent) of an organic substance from an aqueous solution is carried by shaking the aqueous solution with a immiscible organic solvent, like ether in a separating funnel.
• The distribution ratio being in favour of ether, most of
the organic substance passes into ether layer.
• On standing, the aqueous and ether layers separate in the
funnel. The ether layer is then transferred to a distillation flask and distilled so as the organic substance is left as residue in the flask.
• If desired, the process may be repeated with aqueous layer
left after the first extraction with a fresh quantity of the solvent.
• The other common solvents used for extraction are hexane, benzene, chloroform, acetone, carbon disulphide, etc.
• The greater the distribution ratio is in favour of the organic solvent, the greater will be the amount extracted in any one operation
1a. MULTIPLE EXTRACTION
• The process of extraction when carried with the total amount of the given solvent in a single operation, is referred to as simple extraction.
• To recover the maximum amount of the substance from
aqueous solution, the extraction is made in two or more successive operations using small portions of the solvent provided.
• This is called multiple extraction or multi-step extraction.
• In such a process the aqueous solution is first extracted
with a portion of the solvent in a separating funnel.
• The aqueous layer from which some substance has been
removed is then transferred to another funnel.
• This is shaken with a second portion of the solvent.
• Similarly, the aqueous layer from the second extraction is
treated with a third portion of solvent, and so on.
MULTIPLE EXTRACTION IS MORE EFFICIENT
Suppose 100 ml of an aqueous solution contains A grams of an organic substance.
The substance can be extracted with ether, its distribution
ratio being twice in favor of ether.
100 ml of ether is available which may be used in one lot or
in two portions of 50 ml each.
(1) Using all the ether in one lot. Let x grams be the weight of the substance extracted in the solvent layer. Then the amount of substance left in the water layer = A – x grams. Therefore,
Concentration in ether layer = x/100
Concentration in water layer = (A – x)/100
C ether
———– = K
I.e 2
C water
x /100
———– = 2
(A – x) /100
Hence x = 2/3 A
Thus 66% of substance is extracted.
(2) Using two 50 ml portions of ether. Let x1 grams of substance be extracted in the first operation with 50 ml ether. Thus,
Concentration in ether layer = x1/50
Concentration in water layer = (A – x1)/100
C ether
———– = K
I.e 2
C water
x1 / 50
———– = 2
(A – x1) /100
Hence x = 1/2 A
50% of substance is extracted.
II Extraction
The substance left in water layer is ½ A.
Let x2 grams be the substance removed from the water layer when it is extracted with another 50 ml portion of ether. Hence,
Concentration in ether layer = x2/50
Concentration in water layer = 1/2 A – x2
x2 / 50 = 2 (1/ 2A – x2 ) /100
Hence x2 = 1/4 A
25% of substance is extracted.
50%+25% =75%
Thus 75 per cent of substance is extracted by using two 50 ml portions of the solvent as against 66 per cent when 100 ml solvent is used in one lot.
Similarly, by using four 250 ml portions of ether it is possible to extract 80.2% of substance. Five 200 ml portions of ether would likewise remove 83.8%.
But it is not possible to remove the whole of the dissolved
substance, however large the number of extractions may be. Small quantity must always be left behind.
2. PARTITION CHROMATOGRAPHY
• This is a modern technique of separating a mixture of
small amounts of organic materials.
• A paste of the mixture is applied at the top of a column
of silica soaked in water.
• Another immiscible solvent, hexane, is allowed to flow
down the column.
• Each component of the mixture is partitioned between the
stationary liquid phase (water) and the mobile liquid phase (hexane).
• The various components of the mixture are extracted by
hexane in order of their distribution coefficients.
• Thus the component with the highest distribution coefficient is first to move down in the flowing hexane which is collected separately.
• Similarly, a component with a lower distribution ratio comes down later and is received in another vessel.
3. DESILVERIZATION OF LEAD (PARKE’S PROCESS)
• When molten zinc is added to molten lead containing silver
(argentiferous lead), zinc and lead form immiscible layers and silver is distributed between them.
• Since the distribution ratio is about 300 in favour of zinc at 800º C, most of silver passes into the zinc layer.
• On cooling the zinc layer, an alloy of silver and zinc separates.
• The Ag-Zn alloy is distilled in a retort when zinc passes
over leaving silver behind.
• The lead layer still contains unextracted silver. This is
treated with fresh quantities of molten zinc to recover most of the silver.
4. CONFIRMATORY TEST FOR BROMIDE AND IODIDE
• The salt solution is treated with chlorine water. Small
quantity of bromine or iodine is thus liberated.
• The solution is then shaken with trichloromethane (chloroform).
• On standing chloroform forms the lower layer.
• The free bromine or iodine being more soluble in chloroform concentrates into the lower layer, making it red for bromine and violet for iodine.
5. DETERMINATION OF SOLUBILITY
• Suppose the solubility of iodine in benzene is to be determined.
• Iodine is shaken with water and benzene.
• At equilibrium concentrations of iodine in benzene (Cb)
and water (Cw) are found experimentally and the value of distribution coefficient calculated.
Sb/Sw = KD
Where Sb = solubility in benzene; and Sw = solubility in
water
6. DISTRIBUTION INDICATORS
• In iodine titrations, the end point is indicated by adding starch suspension which turns blue.
• A greater sensitivity can be obtained by using ‘Distribution Indicator’.
• A few drops of an immiscible organic solvent such as
chloroform or carbon tetrachloride is added to the solution.
• The bulk of any iodine present passes into the organic layer and imparts intense violet color to it.
7. DEDUCING THE FORMULA OF A COMPLEX ION (I3– )
• Some iodine is added to a solution of KI and the reaction
mixture shaken with benzene.
• The [I2] in water layer can be found knowing the value of
KD (determined separately) and concentration of iodine in benzene (determined by titration against thiosulphate).
• The total concentration of iodine, [I2] + [I3] in water layer is found by titration against thiosulphate
• Knowing [I2] from, [I3] can be calculated
• The initial concentration of KI taken is represented by the equilibrium concentrations
• [I–] + [I3−]. Knowing [I3−], [I–] can be found.
• Substituting the above values of concentrations in the law of Mass Action equation of the reaction in water layer
[I3- ]/ [I2 ][I-] = K
• The value of equilibrium constant K can be calculated.
• If it comes out to be constant for different concentrations of iodine, it stands confirmed that the formula of the complex I3−
9. DETERMINATION OF ASSOCIATION AND DISSOCIATION
ASSOCIATION
DISSOCIATION
Summary
• If two immiscible solvents A and B are taken in a beaker,
they form separate layers. When a solute X which is soluble in both solvents is added, it gets distributed or partitioned between them. Molecules of X pass from solvent A to B and from solvent B to A. Finally a dynamic equilibrium is set up. At equilibrium, the rate, at which molecules of X pass from one solvent to the other is balanced
• Limitations of distribution law include Constant temperature, same molecular state, Equilibrium concentrations, dilute solutions, Non- miscibility of solvents
• Henry’s law states: at a constant temperature the solubility of a gas in a liquid is proportional to the pressure of the gas above it. It is mathematically expressed as C = kP
• Extraction with a solvent
• Partition chromatography
• Desilverization of lead
• Confirmatory test for bromide and iodide
• Determination of solubility
• Distribution indicators
• Deducing the formula of a complex ion
• Determination of association and dissociation
For PDF Notes Click on Download Button